Perimeter Formula: Everything You Need to Know

Introduction

Understanding the perimeter formula is crucial for anyone dealing with geometry, whether you’re a student, a professional, or simply a math enthusiast. The perimeter formula helps you calculate the total distance around a shape, which is essential for various practical applications. In this guide, we’ll explore the perimeter formula in detail, covering its applications for different shapes, examples, and tips to master this fundamental concept.

What is the Perimeter Formula?

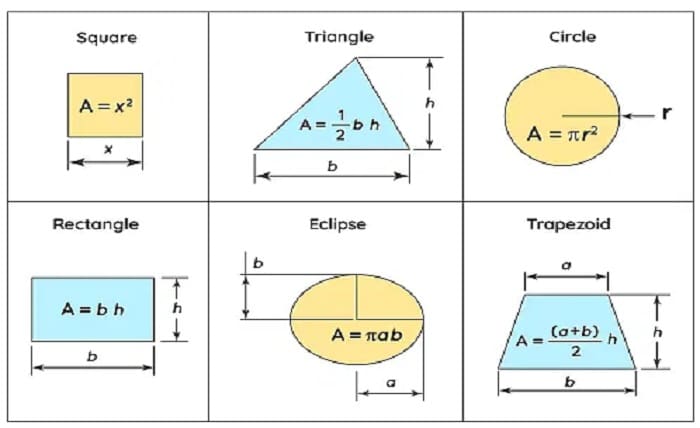
The perimeter formula refers to the mathematical equation used to determine the total length of the boundary of a shape. For different shapes, the perimeter formula varies, but the core idea remains the same: summing up all the sides of the shape. For example, the perimeter formula for a rectangle is $P=2\times (l+w)$, where $l$ is the length and $w$ is the width. Understanding the perimeter formula is essential for solving geometry problems accurately.

Perimeter Formula for Rectangles

For rectangles, the perimeter formula is quite straightforward. To find the perimeter, you simply add up the lengths of all four sides. Since opposite sides of a rectangle are equal, the formula becomes $P=2\times (l+w)$, where $l$ is the length and $w$ is the width. This formula helps in various real-world scenarios, such as determining the amount of fencing required for a rectangular garden or the length of trim needed for a rectangular room.

Perimeter Formula for Squares

The perimeter formula for squares is even simpler. Because all four sides of a square are equal, the perimeter formula is $P=4\times s$, where $s$ is the length of one side. This formula is particularly useful when dealing with squares in design projects, such as tiling a square floor or constructing a square-shaped garden bed. The perimeter formula for squares allows for quick and accurate calculations.

Perimeter Formula for Triangles

Triangles come in various forms, but the perimeter formula remains consistent. To find the perimeter of a triangle, you sum the lengths of all three sides. For example, if a triangle has sides of lengths $a$, $b$, and $c$, the perimeter formula is $P=a+b+c$. This formula is crucial in various applications, including construction, where accurate perimeter measurements are necessary for building materials.

Perimeter Formula for Circles

The perimeter formula for circles is known as the circumference formula. Unlike polygons, circles do not have sides, so the formula is based on the radius or diameter. The circumference $C$ is calculated using the formula $C=2\pi r$, where $r$ is the radius, or $C=\pi d$, where $d$ is the diameter. Understanding the circumference formula is important for tasks such as measuring circular tracks or designing round objects.

Perimeter Formula for Regular Polygons

For regular polygons, where all sides and angles are equal, the perimeter formula is $P=n\times s$, where $n$ is the number of sides and $s$ is the length of one side. This formula applies to shapes like pentagons, hexagons, and octagons. Using the perimeter formula for regular polygons helps in applications such as designing patterns and calculating materials for geometric shapes.

Practical Applications of the Perimeter Formula

The perimeter formula is not just a theoretical concept; it has numerous practical applications. Whether you’re landscaping a garden, building a fence, or creating a piece of artwork, knowing how to use the perimeter formula can save time and resources. For example, calculating the perimeter of a playground or a swimming pool can help in determining the amount of material needed for installation or renovation.

Common Mistakes with the Perimeter Formula

When using the perimeter formula, there are common mistakes that people often make. One common error is forgetting to include all sides in the calculation, especially for irregular shapes. Another mistake is using incorrect units or failing to convert units when necessary. To avoid these pitfalls, always double-check your measurements and ensure you’re using the correct formula for the shape you’re working with.

Tips for Mastering the Perimeter Formula

Mastering the perimeter formula involves practice and a clear understanding of different shapes. Start by working on simple shapes like squares and rectangles before moving on to more complex polygons and circles. Use visual aids and diagrams to help you understand the shape’s dimensions and how they relate to the perimeter formula. Additionally, practicing with real-world problems can enhance your skills and make calculations more intuitive.

Conclusion

The perimeter formula is a fundamental concept in geometry with wide-ranging applications. Whether you’re calculating the boundary of a simple square or a complex polygon, understanding and applying the perimeter formula accurately is essential. By familiarizing yourself with the formulas for various shapes and practicing regularly, you can enhance your mathematical skills and apply these concepts effectively in everyday situations.

FAQs

1. What is the basic perimeter formula for a rectangle?

The basic perimeter formula for a rectangle is $P=2\times (l+w)$, where $l$ is the length and $w$ is the width.

2. How do you calculate the perimeter of a circle?

The perimeter of a circle, known as the circumference, is calculated using the formula $C=2\pi r$, where $r$ is the radius, or $C=\pi d$, where $d$ is the diameter.

3. What is the perimeter formula for a triangle?

The perimeter formula for a triangle is $P=a+b+c$, where $a$, $b$, and $c$ are the lengths of the three sides.

4. How can I apply the perimeter formula in real life?

The perimeter formula can be applied in various real-life scenarios, such as measuring the length of fencing needed for a garden, determining the amount of trim required for a room, or calculating the materials for a geometric design.

5. What are some common mistakes when using the perimeter formula?

Common mistakes include forgetting to include all sides of the shape, using incorrect units, or failing to convert units properly. Always double-check measurements and ensure the correct formula is used for accurate calculations.