Power Rule in Calculus: A Comprehensive Guide

Introduction

The power rule is one of the most fundamental and widely used techniques in calculus for finding derivatives of polynomial functions. Understanding the power rule is essential for anyone studying calculus, as it provides a straightforward method for differentiating functions of the form f(x)=xnf(x) = x^nf(x)=xn, where $n$ is a real number. In this comprehensive guide, we will delve into the power rule, exploring its definition, applications, examples, and common misconceptions.

The power rule is a critical concept that simplifies the process of differentiation, making it easier to solve complex calculus problems. Whether you’re a student, educator, or professional, mastering the power rule is crucial for success in mathematics and related fields.

Definition of the Power Rule

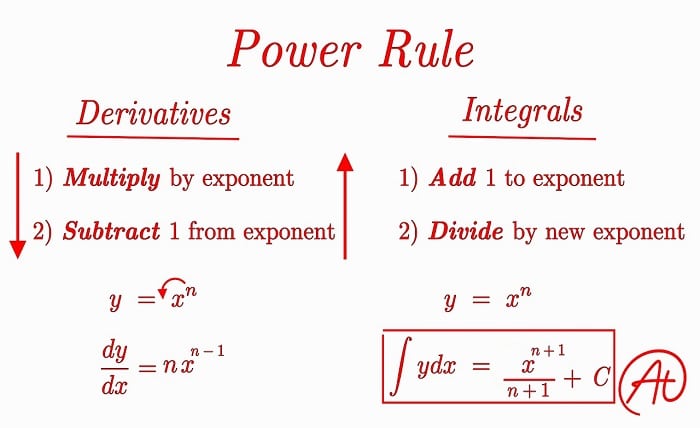
The power rule states that if f(x)=xnf(x) = x^nf(x)=xn, then the derivative of $f(x)$ with respect to $x$ is f′(x)=nxn−1f'(x) = nx^{n-1}f′(x)=nxn−1. This rule applies to any real number $n$, including positive integers, negative integers, and fractions. The power rule is a straightforward and powerful tool that allows for quick differentiation of polynomial functions.

Understanding the power rule involves recognizing the pattern of reducing the exponent by one and multiplying by the original exponent. This simple yet effective technique is the foundation for more advanced differentiation methods in calculus.

Historical Context of the Power Rule

The power rule has its roots in the development of calculus by Sir Isaac Newton and Gottfried Wilhelm Leibniz in the late 17th century. Both mathematicians independently formulated the fundamental principles of calculus, including differentiation and integration. The power rule emerged as a natural consequence of their work on the rates of change and the slopes of curves.

Studying the historical context of the power rule provides valuable insights into its significance and the evolution of mathematical thought. The development of the power rule marked a major advancement in the ability to solve real-world problems using calculus.

Basic Applications of the Power Rule

The power rule is used extensively in various fields to find the derivatives of polynomial functions. Some basic applications of the power rule include solving physics problems involving motion, calculating rates of change in economics, and determining the slopes of curves in geometry. By applying the power rule, we can easily find the instantaneous rate of change of a function, which is essential for analyzing dynamic systems.

In everyday use, the power rule helps simplify complex mathematical expressions, making it easier to solve equations and understand relationships between variables. Mastering the power rule opens up numerous opportunities for practical applications in science, engineering, and economics.

Advanced Applications of the Power Rule

Beyond basic applications, the power rule plays a crucial role in more advanced topics in calculus and mathematical analysis. For example, it is used in the chain rule, product rule, and quotient rule, which are essential for differentiating more complex functions. The power rule also forms the basis for finding higher-order derivatives and solving differential equations.

In fields such as physics, engineering, and finance, the power rule is used to model and analyze systems involving polynomial relationships. Understanding these advanced applications of the power rule is essential for tackling more complex mathematical challenges.

Examples of the Power Rule in Action

To better understand the power rule, let’s consider a few examples:

1. Example 1: Find the derivative of f(x)=x3f(x) = x^3f(x)=x3. Using the power rule, f′(x)=3×3−1=3x2f'(x) = 3x^{3-1} = 3x^2f′(x)=3x3−1=3x2.
2. Example 2: Find the derivative of g(x)=1x2g(x) = \frac{1}{x^2}g(x)=x21​. Rewriting $g(x)$ as x−2x^{-2}x−2, we apply the power rule: g′(x)=−2x−3=−2x3g'(x) = -2x^{-3} = -\frac{2}{x^3}g′(x)=−2x−3=−x32​.
3. Example 3: Find the derivative of h(x)=xh(x) = \sqrt{x}h(x)=x​. Rewriting $h(x)$ as x1/2x^{1/2}x1/2, we apply the power rule: h′(x)=12x−1/2=12xh'(x) = \frac{1}{2}x^{-1/2} = \frac{1}{2\sqrt{x}}h′(x)=21​x−1/2=2x​1​.

These examples demonstrate how the power rule simplifies the process of finding derivatives of various polynomial functions.

Common Mistakes with the Power Rule

While the power rule is straightforward, there are common mistakes that students and practitioners should avoid. One frequent error is misapplying the rule to functions that are not in the form xnx^nxn. Another common mistake is incorrect simplification of the resulting expression after differentiation.

Understanding and recognizing these common mistakes helps ensure accurate application of the power rule. Practice and careful attention to detail are key to mastering this fundamental calculus technique.

Integrating Functions Using the Power Rule

The power rule is not only useful for differentiation but also for integration, which is the reverse process. The integration counterpart to the power rule states that ∫xndx=xn+1n+1+C\int x^n dx = \frac{x^{n+1}}{n+1} + C∫xndx=n+1xn+1​+C, where $C$ is the constant of integration, and n≠−1n \neq -1n=−1.

Understanding how to integrate functions using the power rule is essential for solving problems involving areas under curves and accumulated quantities. Mastery of both differentiation and integration using the power rule provides a strong foundation for further studies in calculus.

The Power Rule in Real-World Applications

The power rule has numerous real-world applications that demonstrate its practical value. In physics, it is used to analyze motion and forces, such as calculating the velocity and acceleration of objects. In economics, the power rule helps determine marginal costs and revenue functions. In engineering, it aids in the design and analysis of systems involving polynomial relationships.

These real-world applications highlight the importance of the power rule in solving practical problems and making informed decisions based on mathematical analysis.

Teaching the Power Rule Effectively

Effective teaching of the power rule involves clear explanations, practical examples, and opportunities for hands-on practice. Educators can use visual aids, such as graphs and diagrams, to illustrate the concept of differentiation and the application of the power rule. Providing students with a variety of problems to solve helps reinforce their understanding and build confidence.

Incorporating technology, such as graphing calculators and computer algebra systems, can also enhance the learning experience by allowing students to explore the power rule in interactive and dynamic ways. Effective teaching strategies ensure that students grasp the fundamental principles and can apply the power rule accurately in different contexts.

Conclusion:

The power rule is a fundamental and versatile tool in calculus that simplifies the process of differentiation for polynomial functions. Understanding the power rule is essential for students and professionals in mathematics, science, engineering, and economics. By mastering the power rule, individuals can solve complex problems, analyze dynamic systems, and make informed decisions based on mathematical principles.

From its historical development to its real-world applications, the power rule remains a cornerstone of calculus. By avoiding common mistakes, practicing with examples, and integrating effective teaching strategies, one can achieve proficiency in using the power rule to tackle a wide range of mathematical challenges.

FAQs

1. What is the power rule in calculus? The power rule is a basic differentiation rule stating that if f(x)=xnf(x) = x^nf(x)=xn, then the derivative is f′(x)=nxn−1f'(x) = nx^{n-1}f′(x)=nxn−1.

2. How is the power rule applied in integration? In integration, the power rule states that ∫xndx=xn+1n+1+C\int x^n dx = \frac{x^{n+1}}{n+1} + C∫xndx=n+1xn+1​+C, where $C$ is the constant of integration, and n≠−1n \neq -1n=−1.

3. What are common mistakes when using the power rule? Common mistakes include misapplying the rule to non-polynomial functions and incorrect simplification of the resulting expressions after differentiation.

4. Can the power rule be used for negative and fractional exponents? Yes, the power rule applies to any real number $n$, including positive integers, negative integers, and fractions.

5. What are some real-world applications of the power rule? The power rule is used in physics to analyze motion, in economics to determine marginal costs, and in engineering to design systems involving polynomial relationships.